Cauchy, A.L.
Mémoire sur les intégrales définies [AND] Mémoire sur la theorie de la propagation des ondes a la surface d'un fluide pesant...
Paris, Academie des Sciences, 1827. 4to (25.5 x 20.0 cm). In a fine contemporary full calf binding. Spine with five raised bands, red and black morocco title vignettes and gilt floral patterns; boards with dentelles; lightly red-speckled edges.
Two large and very important papers by Augustin Louis Cauchy (1789-1857), contained in the first volume of the Mémoires des Savans Étrangers. The most important paper is Cauchy's cornerstone essay on definite integrals. It is essential in the history of mathematics, partly laying the basis for the Cauchy-Riemann equations. "In 1814 he submitted to the French Academy the treatise on definite integrals that was to become the basis of the theory of complex functions. In 1816 he won a prize contest of the French Academy on the propagation of waves at the surface of a liquid; his results are now classics in hydrodynamics." (DSB) Cauchy's method was significant because of his departure from the traditional use of geometry to treat the definite integral. The theory was read to the Academy by Cauchy in 1814, but only published in 1827 in this volume (pp. 599-799) and includes the rapport, introduction, parts 1 and 2, and two supplements. The second paper, on the "propagation des ondes a la surface" is on pp. 3-313. This volume also contains a memoir on the "theorie de la lune" by Damoiseau. Provenance: pictorial bookplate of the Radcliffe Observatory (the astronomical observatory of the University of Oxford from 1773 until 1934) on the front pastedown. Some small, skilful repairs, doing justice to an unusually fine and elegant contemporary binding. DSB III, pp. 137-138.

![Mémoire sur les intégrales définies [AND] Mémoire sur la theorie de la propagation des ondes a la surface d'un fluide pesant...](https://schierenberg.nl/media/cache/product_thumb/49690/49690.jpg)
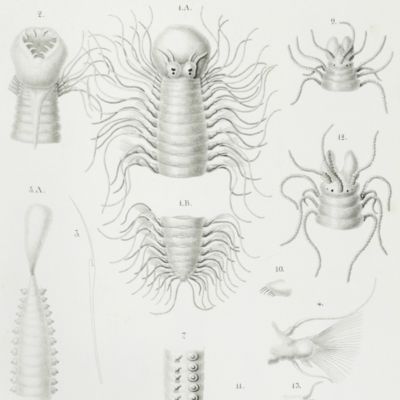
![image for Miscellanea Silurica I-III. I. Über die Russischen Silurischen Leperditien mit Hinzuziehung einiger Arten aus den Nachbarländern (Baltikum); II. Über einige neue Baltisch-Silurische Petrefacten; III. I. Nachtrag zur Monographie der Russischen Silurischen Leperditien [AND] II. Die Crustaceenfauna der Eurypterenschichten von Rootziküll auf Oesel.](https://schierenberg.nl/media/cache/product_thumb/19368/19368_x.jpg)
![image for Malayan Ferns. Handbook to the determination of the ferns of the Malayan Islands (incl. those of the Malay Peninsula, the Philippines and New Guinea). [AND] (Correcting sheet).](https://schierenberg.nl/media/cache/product_thumb/76327/76327_x.jpg)












![image for Revision du nummulitique Égyptien. [AND] Nouvelle contribution à la paléontologie du nummulitique Égyptien. [AND] Étude complémentaire sur la paléontologie du nummulitique Égyptien. [Complete].](https://schierenberg.nl/media/cache/product_thumb/76656/76656_x.jpg)
![image for Histoire naturelle des coquilles contenant leur description, leurs moeurs des animaux qui les habitent et leurs usages. Avec figures dessinées d'apres nature. Tome premier - II - III - IV - V. [Complete].](https://schierenberg.nl/media/cache/product_thumb/75318/75318.jpg)
![image for Naauwkeurige beschryving der aardgewassen, waar in de veelerley aart en bijzondere eigenschappen der boomen, heesters, kruyden, bloemen, met haare vrugten, zaden, wortelen en bollen, neevens derzelver waare voort-teeling, gelukkige aanwinning, en heylzaame genees-krachten, na een veel-jarige oeffening en eigen ondervinding, in drie onderscheide boeken, naauwkeuriglijk beschreeven worden; foor den heer Abraham Munting, In zijn leeven, hoogleraar der genees- en kruydkunde in de vermaarde Akademie te Groeningen. Nu eerst nieuwelijks uitgegeeven, en met meer dan 250 afbeeldingen, all naer 't leeven geteekend en konstiglijk in 't koper gesneeden, vercierd. Met nodige registers verrijkt. [Nauwkeurige beschryving der aard-gewassen].](https://schierenberg.nl/media/cache/product_thumb/70899/70899_x.jpg)
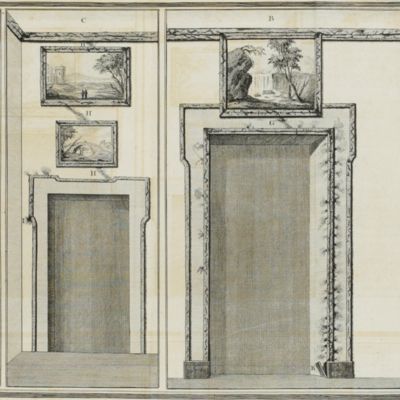
![image for Tapisserie de haute-lisse des Gobelins [BOUND WITH] Tapisserie de basse-lisse des Gobelins.](https://schierenberg.nl/media/cache/product_thumb/51981/51981_x.jpg)


![image for Les récents voyages des Néerlandais à la Nouvelle-Guinée. [AND] Les derniers voyages des Néerlandais à la Nouvelle-Guinée.](https://schierenberg.nl/media/cache/product_thumb/31793/31793_x.jpg)



![image for Spermatologiae vegetabilis. Pars I. De seminum existentia differentia et usu. Pars II. De seminum ortu foecundatione et incremento. Pars III. De seminum collectione duratione et conservatione. Pars IV. De seminum ad sementem praeparatione. Pars V. De seminum satione. Pars VI. De germinationis adminiculis. Pars VII et ultima. De germinatione. [Complete].](https://schierenberg.nl/media/cache/product_thumb/32304/32304.jpg)

![image for Illustrations of the zoology of South Africa; consisting chiefly of figures and descriptions of the objects of natural history collected during an expedition into the interior of South Africa, in the years 1834, 1835, and 1836; fitted out by "The Cape of Good Hope Association for Exploring Central Africa". Published under the authority of the Lords Commissioners of Her Majesty's Treasury. Mammalia. Aves. Reptilia. Pisces. Invertebratae. [Complete].](https://schierenberg.nl/media/cache/product_thumb/74777/74777_x.jpg)
![image for Giant louse [from the Encyclopédie ou Dictionnaire des Sciences, plate 84].](https://schierenberg.nl/media/cache/product_thumb/72386/72386_x.jpg)
![image for Des Ritters Carl von Linné vollständiges Pflanzensystem nach der dreyzehnten lateinischen Ausgabe und nach Anleitung des holländischen houtteuynischen Werks übersetzt und mit einer ausführlichen erklärung ausgefertiget. Erster - Eilfter Theil. [Complete].](https://schierenberg.nl/media/cache/product_thumb/68492/68492_x.jpg)


![image for Scientific Results of the Vernay-Lang Kalahari Expedition, March to September 1930. The Freshwater fishes. [AND] Reptilia and Amphibia.](https://schierenberg.nl/media/cache/product_thumb/68901/68901.jpg)
![image for Botanica Pharmaceutica exhibens plantas officinalis quarum nomina indispensatoris recentur, cum Iconibus… Plate 222. <em> Viscum album.</em> [Mistletoe].<em></em>](https://schierenberg.nl/media/cache/product_thumb/73477/73477_x.jpg)
![image for [18th-century floral design - daffodils and butterfly]](https://schierenberg.nl/media/cache/product_thumb/69812/69812_x.jpg)





![image for [18th-century floral design - moons and flowers]](https://schierenberg.nl/media/cache/product_thumb/69810/69810_x.jpg)


![image for Diagnoses algarum novarum. Post sylloges editionem descriptarum. I (Centuriae I-XI) [AND] II (Centuria I). [All published].](https://schierenberg.nl/media/cache/product_thumb/46582/46582_x.jpg)





![image for Mission au Tibesti (1930-1931) dirigée par M. Dalloni. I-II. [Complete].](https://schierenberg.nl/media/cache/product_thumb/13269/13269_x.jpg)






![image for De Bare'e sprekende Toradjas van Midden-Celebes. [Complete, in four volumes].](https://schierenberg.nl/media/cache/product_thumb/15672/15672_x.jpg)

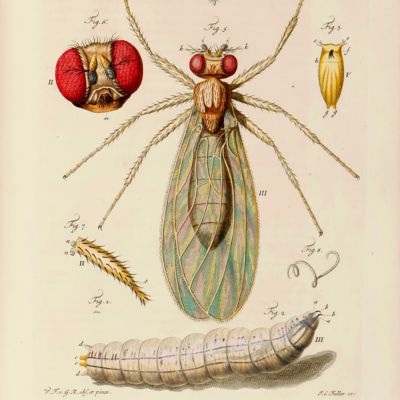
![image for Über einige Anwendungen des Saitengalvanometers. [AND] Über eine neue Methode zur Dämpfung oszillierender Galvanometerausschläge" [AND] Weiter Mitteilungen über das Saitengalvanometer. Analyse der saitengalvanometrischen Kurven. Masse und Spannung des Quarzfadens und Widerstand gegen die Fadenbewegung.](https://schierenberg.nl/media/cache/product_thumb/66174/66174_x.jpg)

![image for The butterflies of Malacca. [AND] On <em>Hypsiprymnodon</em>, Ramsay, a genus indicative of a distinct family (Pleopodidae) in the diprodont section of the marsupialia.](https://schierenberg.nl/media/cache/product_thumb/73535/73535_x.jpg)

![image for A History of British Fishes. [Large paper copy].](https://schierenberg.nl/media/cache/product_thumb/47963/47963.jpg)
![image for Astronomische Untersuchungen. Erster Band [AND] Zweiter Band. [Complete].](https://schierenberg.nl/media/cache/product_thumb/63633/63633.jpg)
![image for Revision of Indian fossil plants. Part I. Coniferales (a. Impressions and incrustations). [AND] Part II (b. Petrifactions).](https://schierenberg.nl/media/cache/product_thumb/32148/32148.jpg)


![image for [18th-century floral design - carnations]](https://schierenberg.nl/media/cache/product_thumb/69811/69811_x.jpg)

![image for [Portrait by Jules Boilly]](https://schierenberg.nl/media/cache/product_thumb/77469/77469_x.jpg)


![image for Fauna und Flora des Golfes von Neapel und der angrenzenden Meeres-Abschnitte herausgegeben von de zoologischen Station zu Neapel. 33. Monographie: Stomatopoden. Erster Theil. [All published].](https://schierenberg.nl/media/cache/product_thumb/71905/71905_x.jpg)




![image for Kunstformen der Natur. Plate 23. <em>Cristatella</em> - Bryozoa - Moostiere [Bryozoa]](https://schierenberg.nl/media/cache/product_thumb/70073/70073_x.jpg)
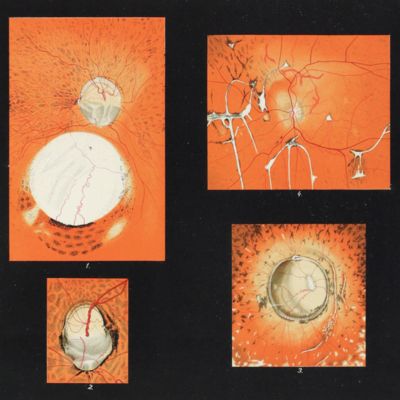
![image for System der Polydesmiden. I Theil - II Theil. [Bound copies; complete].](https://schierenberg.nl/media/cache/product_thumb/71288/71288_x.jpg)
![image for Natuur-en Geneeskundig Archief van Nederlands Indie. Volumes I-IV. [All published].](https://schierenberg.nl/media/cache/product_thumb/49096/49096_x.jpg)

![image for Histoire naturelle des poissons de la France. Avec 220 figures dessinées d'après nature. I, II, III [AND] Histoire naturelle des poissons de la France. Supplément. Avec 7 figures dans le texte. [Complete].](https://schierenberg.nl/media/cache/product_thumb/16534/16534.jpg)
![image for Mille et un livres botaniques de la collection Arpad Plesch. [Limited series copy, with handwritten dedication by the author].](https://schierenberg.nl/media/cache/product_thumb/77456/77456_x.jpg)
![image for Report of the First Scientific Expedition to Manchoukuo. Under the leadership of Shigeyasu Tokunaka. June - October 1933. Section II [Geology]. Part I-IV. [Complete].](https://schierenberg.nl/media/cache/product_thumb/51507/51507.jpg)




![image for Ueber den <em>Archegosaurus</em> der Steinkohlenformation [AND] Fische, Echinodermen und andere Versteinerungen aus dem Muschelkalk Oberschlesiens [AND] <em>Sphyraenodus</em> aus dem Tertiärsande von Flonheim.](https://schierenberg.nl/media/cache/product_thumb/64672/64672.jpg)

![image for Tijdschrift van het Aardrijkskundig Genootschap, gevestigd te Amsterdam. Eerste Deel [Journal of the Geographical Society in Amsterdam, Volume 1].](https://schierenberg.nl/media/cache/product_thumb/67342/67342.jpg)

![image for Mongolian horsemen with falcons, cats and rabbits. [Eight original paintings on silk].](https://schierenberg.nl/media/cache/product_thumb/72052/72052_x.jpg)
![image for Ophiures recueillis par l'Investigator dans l'Océan Indien. I. Les ophiures de mer profondes [AND] Ophiures recueillis par l'Investigator dans l'Océan Indien II. Les ophiures littorales.](https://schierenberg.nl/media/cache/product_thumb/60990/60990_x.jpg)
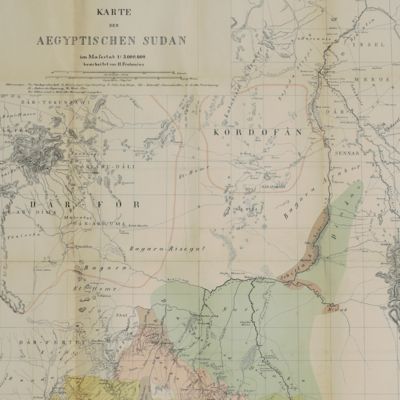
![image for Geognostische Karte von Württemberg [AND] Begleitworte zur geognostischen Specialkarte von Württemberg.](https://schierenberg.nl/media/cache/product_thumb/28715/28715.jpg)






![image for Anatomisch-Physiologische Untersuchungen der Insekten und Krustenthiere. Ersten Bandes erstes Heft. [All published].](https://schierenberg.nl/media/cache/product_thumb/60195/60195.jpg)

![image for Bulletins de la Société royale de Botanique de Belgique. Volumes 1 - 25. [Bulletin].](https://schierenberg.nl/media/cache/product_thumb/74339/74339_x.jpg)


![image for Documents decoratifs. Planche 54. [Female alphabet]](https://schierenberg.nl/media/cache/product_thumb/70603/70603_x.jpg)
![image for Nursehound Shark [Very large print, framed].](https://schierenberg.nl/media/cache/product_thumb/69872/69872_x.jpg)

![image for Histoire naturelle des singes, peints d'après nature. [AND] Histoire naturelle [des singes et] des makis. [Complete].](https://schierenberg.nl/media/cache/product_thumb/71213/71213_x.jpg)

![image for A catalogue of the Miridae of the World. Catálogo dos mirídeos do mundo. I-V. [Complete].](https://schierenberg.nl/media/cache/product_thumb/76625/76625.jpg)
![image for I miceti patogeni dell'uomo e degli animali, descritti, delineati e preparati per l'osservazione al microscopio, con notizie sopra i rimedi per combatterli. Fasciculo I-X. [Complete].](https://schierenberg.nl/media/cache/product_thumb/67970/67970_x.jpg)
![image for Insects [from the Encyclopédie ou Dictionnaire des sciences, plates 75-83].](https://schierenberg.nl/media/cache/product_thumb/72397/72397_x.jpg)


![image for Fauna der Gaskohle und der Kalksteine der Permformation Böhmens. Band I-IV. [Complete].](https://schierenberg.nl/media/cache/product_thumb/67746/67746_x.jpg)

![image for Les mollusques marins testacés du Maroc. Catalogue non critique. I. Les gastéropodes. [AND] II. Les lamellibranches et les scaphopodes. [AND] Premier supplement.](https://schierenberg.nl/media/cache/product_thumb/74966/74966_x.jpg)

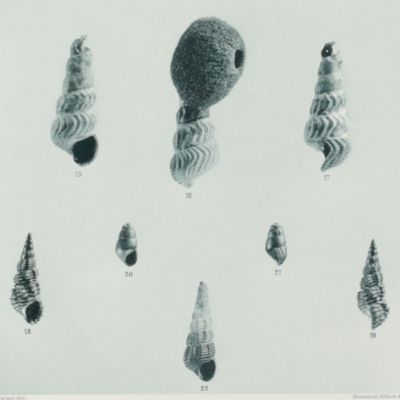


![image for Catalogue of Index of Foraminifera. 1-3. [Complete].](/images/notfound.png)

![image for [Venus Transit]. Mémoires de l'Académie des Sciences de l'Institut de France Tome XLI renfermant les mémoires, rapports et documents relatifs a l'observation du passage de Vénus sur le soleil.](https://schierenberg.nl/media/cache/product_thumb/68662/68662_x.jpg)



![image for Blackheaded pheasant. Male [From: <em>Illustrations of Indian Zoology. Chiefly selected from the collection of Major-General Hardwicke. F.R.S.</em>].](https://schierenberg.nl/media/cache/product_thumb/77269/77269_x.jpg)

![image for Mémoires de la Société Linnéenne de Normandie. Volumes I-V. [IINCLUDING Société Linnéenne de Calvados].](https://schierenberg.nl/media/cache/product_thumb/36283/36283.jpg)
![image for Traité de Paléontologie où histoire naturelle des animaux fossiles considérés dans leurs rapports zoologiques et géologiques. [Text and atlas, complete].](https://schierenberg.nl/media/cache/product_thumb/74907/74907_x.jpg)



![image for Aux amateurs de grands vins de Champagne en Belgique. [AND] An original drawing by Massonet.](https://schierenberg.nl/media/cache/product_thumb/71386/71386_x.jpg)

![image for Saito Ho-On Kai. (The Saito Gratitude Foundation) Monographs 1 - 5. [AND] Reprints.](https://schierenberg.nl/media/cache/product_thumb/73805/73805.jpg)

![image for Histoire générale des voyages, ou nouvelle collection des toutes les relations de voyages par mer et par terre, qui ont été publiées jusq'à présent dans les différentes langues de toutes les nations connus: ... Tome dix-septième. Histoire générale des voyages, depuis le commencement du XVme siècle, dix-septième partie. Livre septième. Vies des gouverneurs généraux, avec l'abregé de l'histoire des etablissemens Hollandois aux Indes Orientales [AND] Histoire naturelle des Indes Orientales.](https://schierenberg.nl/media/cache/product_thumb/62296/62296.jpg)


![image for De Indische Bij, Tijdschrift ter Bevordering van de Kennis der Nederlandsche Volkplantingen en Derzelven Belangen. Eerste Deel. [Volume I; All published].](https://schierenberg.nl/media/cache/product_thumb/43404/43404_x.jpg)
![image for l'Horticulteur Belge, Journal des Jardiniers et Amateurs. Volumes 1-5. [All published].](https://schierenberg.nl/media/cache/product_thumb/43978/43978.jpg)
![image for Encyclopédie méthodique. Dictionnaire des jeux, faisant suite au tome III des mathématiques. [AND] Dictionnaire des jeux familiers, ou des amusemens de société; faisant suite au dictionnaire des jeux, annexé au tome III des mathématiques. [AND] Dictionnaire des jeux mathematiques, contenant l'analyse, les recherches, les calculs, les probabilités & les tables numériques, publiés par plusiers célèbres mathématiciens, relativement aux jeux de hasard & combinaisions; et suite du dictionnaire des jeux.](https://schierenberg.nl/media/cache/product_thumb/68184/68184_x.jpg)

![image for The Norwegian forms of <em>Lithothamnion</em> [AND] New or critical Lithothamnia.](https://schierenberg.nl/media/cache/product_thumb/29446/29446.jpg)
![image for Giant lizard, snake, small lizard and bird [Plate 99 of Seba's <em>Locupletissimi rerum naturalium thesauri accurata descriptio</em>].](https://schierenberg.nl/media/cache/product_thumb/72404/72404_x.jpg)
![image for Arbeiten aus der chirurgischen Klinik der Königlichen Universität Berlin. Erster Theil - Tritter Theil. [Complete].](https://schierenberg.nl/media/cache/product_thumb/66471/66471_x.jpg)
![image for [Original engraved print]](https://schierenberg.nl/media/cache/product_thumb/70154/70154_x.jpg)
![image for Les pédiculines. Essai monographique. I (texte), II (atlas), supplement. [Complete].](https://schierenberg.nl/media/cache/product_thumb/37627/37627.jpg)


![image for Etudes sur la flore du Katanga. [Complete in four parts].](https://schierenberg.nl/media/cache/product_thumb/40048/40048_x.jpg)
![image for Report on the scientific results of the voyage of H.M.S. Challenger during the years 1873-76 under the command of captain George S. Nares, R.N., F.R.S. and the late captain Frank Tourle Thomson, R.N. prepared under the superintendence of the late Sir C. Wyville Thomson, Knt., F.R.S., &c. and now of John Murray. Volumes I - II. Narrative of the Cruise. [The complete narrative].](https://schierenberg.nl/media/cache/product_thumb/50255/50255_x.jpg)
![image for De Bare'e sprekende Toradjas van Midden-Celebes. Tweede, geheel omgewerkte druk. [2nd, revised edition. Complete, in five volumes].](https://schierenberg.nl/media/cache/product_thumb/75785/75785_x.jpg)

![image for [BINDING]. Exercitatio academica ex antiquitatibus Germanicis et Francicis de Butigulariis, praecipue iis qui Norimbergae olim floruerunt quam sub praesidio Christiani Gotlib. Schwarzii com. palat. caes. et prof. publ. a.d. viii decembr a.r.s. mdccxxiii in academico disputantium circulo as disquirendum proponit a. et r. Iohannes Hieronymus Braun. Norimbergensis.](https://schierenberg.nl/media/cache/product_thumb/48874/48874_x.jpg)
![image for Kunstformen der Natur. Plate 64. <em>Caulerpa</em> - Siphoneae - Riesen-Algetten [calcareous algae]](https://schierenberg.nl/media/cache/product_thumb/70157/70157_x.jpg)


![image for Landwirtschaftliche Tafeln. XII. Forstwirtschaft (Holzzucht). [AND] XIII. Forstwirtschaft (Forstbenützung).](https://schierenberg.nl/media/cache/product_thumb/68754/68754_x.jpg)






